
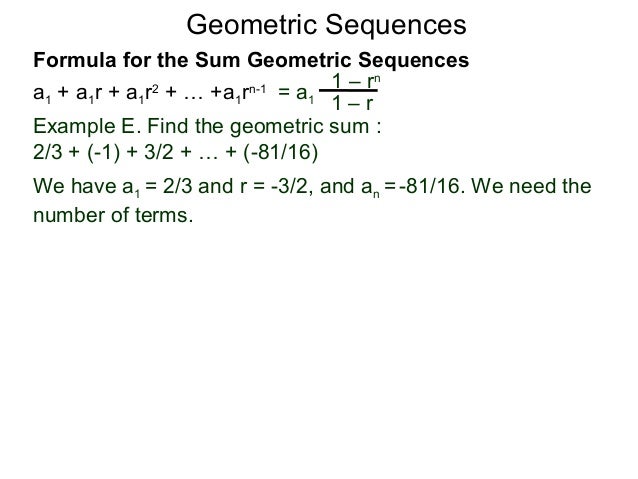
$100 in at the end of a year, or exactly a year later it'd be $105. It's very hard to find a bank account that will actually give Is always willing to give us 5% per year, which is pretty good. So, let's say this is the year, and we're gonna thinkĪbout how much we have at the beginning of the year, and then this is theĭollars in our account. We keep depositing, let's say, $1,000 a year in a bank account. Understand that I'm gonna construct a little bit of a table to understand how our money could grow if
Video we're gonna study geometric series, and to You forgot that every year he deposits $1000 and the money from the previous years collect more and more interest as the years go by. Note that the highest exponent is 2 which is one less than the year number. basically, the first hundred now has been multiplied by 1.05 twice. In the third year the first $1000 that he deposited that had turned into $1050, gains %5 interest and is again multiplied by 1.05. But since he is depositing $1000 every year, in the second year he has a total of $2050, since he has not yet earned interest on the second $1000 that he deposited. In the second year, he gains 5% interest on the $1000, and now it becomes $1050. However, the $1000 deposited in previous years is still earning interest.įor example, in the first year, he deposits $1000.


 0 kommentar(er)
0 kommentar(er)
